Solved Examples and Worksheet for Graphing and Analyzing Quadratic Functions

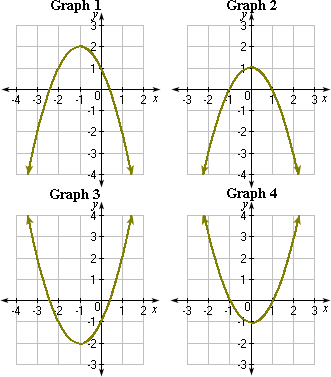
A. Graph 2; opens up;
B. Graph 1; opens up;
Step: 1
Make a table of ordered pairs for the given function.
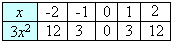
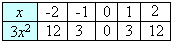
Step: 2
Plot these points on the coordinate plane and connect the points with a smooth curve.
Step: 3
The graph looks like the one below:
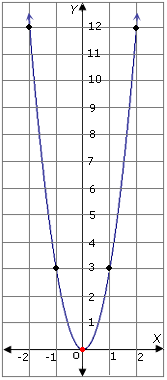

Step: 4
It can be observed from the graph that the parabola opens upward.
Step: 5
The equation of the axis of symmetry is: x
Step: 6
The vertex is at (0, 0).
Step: 7
The parabola opens up. So the vertex is the minimum point.
Correct Answer is : Graph 2; opens up; x
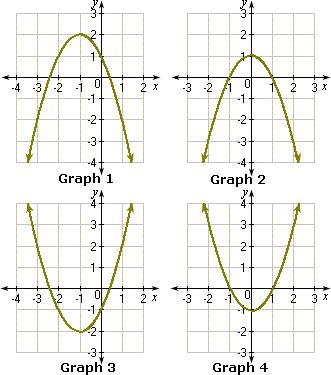
A. Graph 2
B. Graph 1
C. Graph 3
D. Graph 4
Step: 1
[Original quadratic function.]
Step: 2
[Comparing with y ax bx c
Step: 3
The x - b 2 a - 0 2 ( - 1 )
Step: 4
The values of y x x x
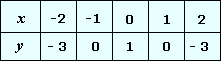

Step: 5
Plot the points and join them with a smooth curve as shown.


Step: 6
The graph matches with Graph 2.
Correct Answer is : Graph 2
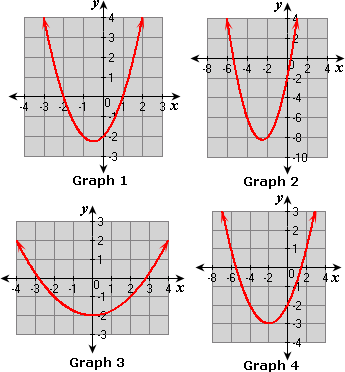
A. Graph 1
B. Graph 2
C. Graph 3
D. Graph 4
Step: 1
[Original quadratic function.]
Step: 2
[Comparing with y ax bx c
Step: 3
The x - b 2 a - 0 2 ( 1 4 )
Step: 4
The values of y x 2 4 x x


Step: 5
Graph 3 satisfies the above table.
Correct Answer is : Graph 3
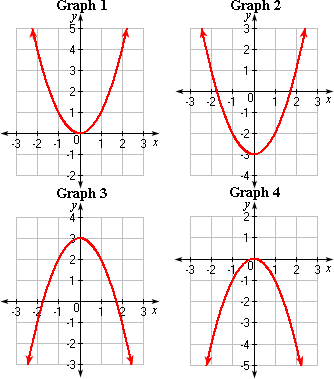
A. Graph 2
B. Graph 1
C. Graph 3
D. Graph 4
Step: 1
[Original function.]
Step: 2
[Comparing with y ax bx c
Step: 3
The x - b 2 a - 0 2 ( - 1 )
Step: 4
The values of y x x x


Step: 5
Plot the points and join them with a smooth curve as shown.

Step: 6
The graph matches with Graph 4.
Correct Answer is : Graph 4
A. Graph 2
B. Graph 1
C. Graph 3
D. Graph 4
Step: 1
[Original quadratic function.]
Step: 2
[Comparing with y ax bx c
Step: 3
The x - b 2 a - 0 2 ( 1 )
Step: 4
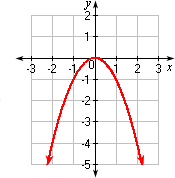
The values of y x x x
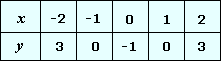

Step: 5
Plot the points and join them with a smooth curve as shown.
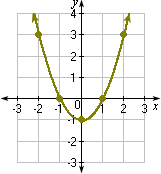

Step: 6
The graph matches with Graph 4.
Correct Answer is : Graph 4
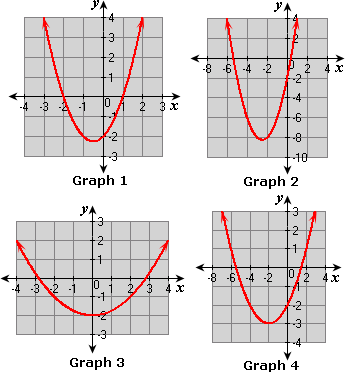
A. Graph 1
B. Graph 3
C. Graph 2
D. Graph 4
Step: 1
[Original quadratic function.]
Step: 2
[Comparing with y ax bx c
Step: 3
The x - b 2 a - 5 2 ( 1 )
Step: 4
The values of y x x x x


Step: 5
Graph 2 satisfies the above table.
Correct Answer is : Graph 2
- Determining a given Relation is a Function-Algebra1-Solved Examples
- Finding Values of a Function for a given Domain-Algebra1-Solved Examples
- Graphing Linear Functions for a Given Domain-Algebra1-Solved Examples
- Graphing Linear Functions-Algebra1-Solved Examples
- Finding the Rate of Change-Algebra1-Solved Examples
- Finding Intercepts of a Linear Equation-Algebra1-Solved Examples
- Graphing Piecewise and Step Functions-Algebra1-Solved Examples
- Graphing Absolute Value Functions-Algebra1-Solved Examples
- Finding Intercepts of Quadratic Functions-Algebra1-Solved Examples
- Identifying and Graphing Square Root Functions-Algebra1-Solved Examples
- Comparing Properties of Two Functions-Algebra1-Solved Examples
- Finding Intervals for Increasing and Decreasing Functions-Algebra1-Solved Examples
- Representing Exponential Functions Using Tables or Graphs-Algebra1-Solved Examples
- Graphing Logarithmic Functions-Algebra1-Solved Examples
- Graphing Trigonometric Functions-Algebra1-Solved Examples
- Identifying and Using Recursive Formula-Algebra1-Solved Examples
Related Worksheet
- Quadratic Function