Solved Examples and Worksheet for Perimeter and Area of Composite Figures

Q1Find the area of the figure.


A. 22 m2
B. 18 m2
C. 25 m2
D. 16 m2

Solutions
Share 
Step: 1
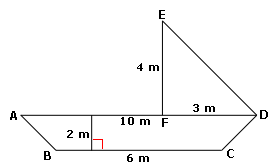
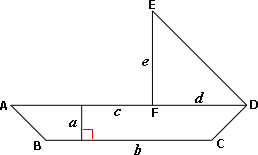
The area of the figure = Area of the trapezoid ABCD + Area of the Δ DEF.
Step: 2
The area of the trapezoid ABCD = 12 x height x (sum of the measures of the parallel sides)
Step: 3
= 12 x height x (AD + BC)
  
Step: 4
= 12 x 2 x (10 + 6)
  [Substitute AD = 10 m, BC = 6 m and height = 2 m.]
Step: 5
= 12 x 2 x 16 = 16 m2
  [Simplify.]
Step: 6
= 16 m2
  [Simplify.]
Step: 7
The area of ΔDEF= 12 x base x height = 12 x FD x EF
Step: 8
= 12 x 3 x 4
  [Substitute FD = 3 m and EF = 4 m.]
Step: 9
= 122 = 6 m2
  [Simplify.]
Step: 10
So, area of the figure = 16 + 6 = 22 m2
  [Substitute the values.]
Correct Answer is :   22 m2
Q2What is the total area of the figure? [Assume that CDEF is a trapezoid.]


A. 5 m2
B. 12 m2
C. 13 m2
D. None of the above

Solutions
Share 
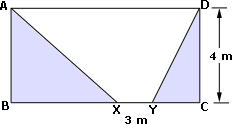
Step: 1
The total area of the figure = area of the triangle ABC + area of the trapezoid CDEF.
Step: 2
The area of the triangle ABC = 12 × base × height
Step: 3
= (12) × BC × AO
  
Step: 4
= (12) × 3 × 2
  [Substitute BC = 3 m and AO = 2 m.]
Step: 5
= 3 m 2
  [Simplify.]
Step: 6
The area of the trapezoid CDEF = (12) × height × (sum of the measures of the parallel sides)
Step: 7
= (12) × CS × (CF + DE)
  
Step: 8
= (12) × 2 × (4 + 6)
  [Substitute CS = 2, CF = 4 and DE = 6.]
Step: 9
= (12) × 2 × 10
  [Work inside the grouping symbols.]
Step: 10
= 10 m2
  [Simplify.]
Step: 11
The total area of the figure = 3 + 10 = 13 m2.
  [Substitute the values.]
Correct Answer is :   13 m2
Q3Find the area of the figure shown.

A. 99 in2
B. 36 in2
C. 72 in2
D. 63 in2

Solutions
Share 
Step: 1

Step: 2
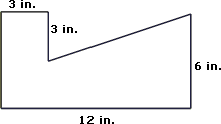
Area of the figure = area of square ABCD + area of rectangle DEFG + area of triangle CGH
Step: 3
= AB × AB + DE × EF + 12× CG × GH
Step: 4
= 3 × 3 + 3 × 12 + 12× 9 × 3
Step: 5
= 9 + 36 + 13.5
Step: 6
= 58.5 in2
Correct Answer is :   72 in2
Q4Find the area of the figure. [Given a = 4 in., b = 12 in., c = 16 in., d = 6 in. and e = 8 in..]


A. 160 in.2
B. 180 in.2
C. 80 in.2
D. 84 in.2

Solutions
Share 
Step: 1
The area of the figure = Area of the trapezoid ABCD + Area of the Δ DEF.
Step: 2
The area of the trapezoid ABCD = 12 × height × (sum of the measures of the parallel sides)
Step: 3
= 12 × height × (AD + BC)
  
Step: 4
= 12 × 4 × (16 + 12)
  [Substitute AD = 16 in., BC = 12 in. and height = 4 in..]
Step: 5
= 12 × 4 × 28 = 56 in.2
  [Simplify.]
Step: 6
The area of ΔDEF = 12 × base × height = 12 × FD × EF
Step: 7
= 12 × 6 × 8
  [Substitute FD = 6 in. and EF = 8 in..]
Step: 8
= 482 = 24 in.2
  [Simplify.]
Step: 9
So, area of the figure = 24 + 56 = 80 in.2
  [Substitute the values.]
Correct Answer is :   80 in.2
Q5Find the area of the figure ABCDE if the area of ABCE is 40 cm2 and area of ECD is 20 cm2.

A. 40 cm2
B. 60 cm2
C. 20 cm2
D. 80 cm2

Solutions
Share 
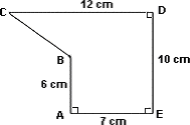
Step: 1
In the given figure, the area of ABCE is 40 cm2 and the area of ECD is 20 cm2.
  [Given.]
Step: 2
The area of ABCDE = area of ABCE - area of ECD
  [From the given figure.]
Step: 3
= 40 - 20
  [From step 1.]
Step: 4
= 20
  [Subtract.]
Step: 5
Therefore, the area of the given figure ABCDE is 20 cm2 .
Correct Answer is :   20 cm2
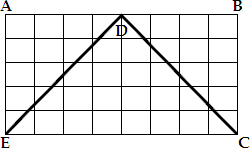
Q6Find the area of rectangle ABCD, if the area of the triangle ABC is 12 cm 2.

A. 24 cm.2
B. 28 cm.2
C. 12 cm.2
D. 16 cm.2

Solutions
Share 
Step: 1
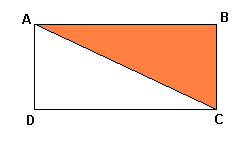
Diagonal AC divides the rectangle into two congruent triangles.
Step: 2
Area of the triangle ABC = 12 cm2.
Step: 3
Area of the rectangle ABCD = 2 × area of traingle ABC.
= 2 × 12
  
Step: 4
So, the area of the rectangle ABCD = 24 cm2.
Correct Answer is :   24 cm.2
Q7Find the area of the figure.


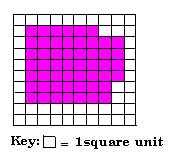
A. 56 square units
B. 48 square units
C. 44 square units
D. 52 square units

Solutions
Share 
Step: 1
In the given figure, 52 squares are colored.
Step: 2
Area of each square = 1 square unit.
Step: 3
Area of 52 squares = 52 × 1 = 52 square units.
Correct Answer is :   52 square units
Q8Find the area of the figure.


A. 38 yd2
B. 27 yd
C. 38 yd
D. 32 yd2

Solutions
Share 
Step: 1

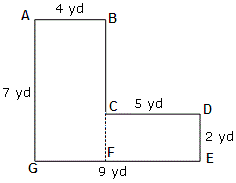
Step: 2
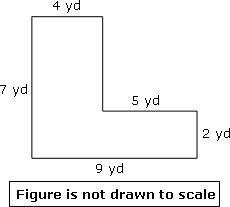
From the figure, Area of the rectangle ABFG = length × width = AG × AB = 7 × 4 = 28 sq.yd
Step: 3
Area of the rectangle CDEF = length × width = CD × DE = 5 × 2 = 10 sq.yd
Step: 4
Total area of the figure ABCDEFG = area of the rectangle ABFG + area of the rectangle CDEF
Step: 5
= 28 + 10 = 38
Step: 6
So, total area = 38 sq.yd
Correct Answer is :   38 yd2
Q9Find the perimeter of the figure.


A. 39 m
B. 39 m2
C. 38 m
D. 38 m2

Solutions
Share 
Step: 1

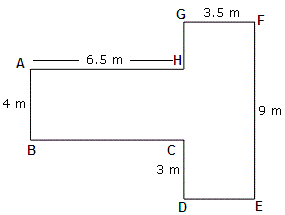
Step: 2
From the figure, AB = 4 m., BC = 6.5 m., CD = 3 m., DE = 3.5 m., EF = 9 m., FG = 3.5 m, GH = 2 m, HA = 6.5 m.
  [GH = FE - (CD + AB).]
Step: 3
Perimeter of the figure = Sum of all the sides of the figure.
  [Formula.]
Step: 4
Perimeter of the figure = AB + BC + CD + DE + EF + FG + GH + HA
Step: 5
4 + 6.5 + 3 + 3.5 + 9 + 3.5 + 2 + 6.5 = 38
  [Substitute the values and add.]
Step: 6
Perimeter of the figure = 38 m.
Correct Answer is :   38 m
Q10Find the perimeter of the figure.

A. 80 cm
B. 47 cm
C. 74 cm
D. 56 cm

Solutions
Share 
Step: 1

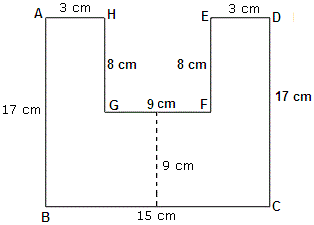
Step: 2
From the figure, AB = 17 m, BC = 15 m, CD = 17 m, DE = 3 m, EF = 8 m, FG = 9 m, GH = 8 m, HA = 3 m
Step: 3
Perimeter of the figure = Sum of all the sides of the figure.
  [Formula.]
Step: 4
Perimeter of the figure = AB + BC + CD + DE + EF + FG + GH + HA
Step: 5
17 + 15 + 17 + 3 + 8 + 9 + 8 + 3 = 80 m
  [Substitute the values and add.]
Step: 6
Perimeter of the figure = 80 m.
Correct Answer is :   80 cm
Q11Find the area of the figure.

A. 88 sq ft
B. 69 sq ft
C. 108 sq ft
D. 105 sq ft

Solutions
Share 
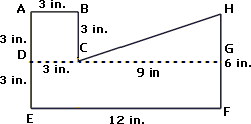
Step: 1
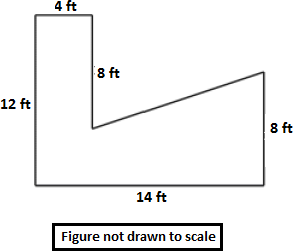
Label the given figure as shown below and draw line EH perpendicular to DF.
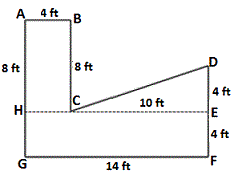
Step: 2
Area of rectangle ABCH = 8 × 4 = 32 sq ft.
  [Area of rectangle = length × width.]
Step: 3
Area of triangle CDE = 12 × 10 × 4 = 20 sq ft.
  [Area of triangle = 12 × base × height.]
Step: 4
Area of rectangle EFGH = 14 × 4 = 56 sq ft.
  [Area of rectangle = length × width.]
Step: 5
The total area of the given figure ABCDEFGH = Area of rectangle ABCH + Area of triangle CDE + Area of rectangle EFGH.
Step: 6
= 32 + 20 + 56
  [From steps 2, 3, and 4.]
Step: 7
= 108
  [Add.]
Step: 8
Therefore, the total area of the given figure is 108 sq ft.
Correct Answer is :   108 sq ft
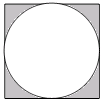
Q12A circle is inscribed in a square of side length 15 cm. What is the area of the shaded region of the square? [Round the answer to the nearest tenth, use π = 3.14]


A. 47.4 cm
B. 50.4 cm2
C. 49.4 cm2
D. 48.4 cm2

Solutions
Share 
Step: 1
From the figure, diameter of the circle = side of the square = 15 cm.
Step: 2
Area of the circle = π(d2)2
  [Radius = diameter2]
Step: 3
= 3.14 x (152)2
  [Substitute the values.]
Step: 4
= 3.14 x (7.5)2
  [Divide 15 by 2.]
Step: 5
= 3.14 x 7.5 x 7.5
Step: 6
= 176.63
  [Multiply.]
Step: 7
Area of the circle = 176.63 = 176.6 cm2
Step: 8
Area of the square = side x side
  [Formula.]
Step: 9
= 15 x 15
  [Substitute the values.]
Step: 10
= 225
  [Multiply.]
Step: 11
Area of the square = 225 cm2
Step: 12
Area of the shaded region = Area of the square - Area of the circle
Step: 13
= 225 - 176.6
  [Substitute the values.]
Step: 14
= 48.4
  [Subtract.]
Step: 15
The area of the shaded region in the figure is 48.4 cm2.
Correct Answer is :   48.4 cm2
Q13What is the area of the shaded region in the figure, if the area of the rectangle ABCD is 48 m2?


A. 20 m2
B. 22 m2
C. 18 m2
D. 16 m2

Solutions
Share 
Q14What is the total area of the figure shown?


A. 23 ft2
B. 14 ft2
C. 15 ft2
D. 11 ft2

Solutions
Share 
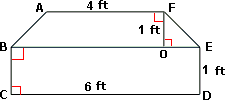
Step: 1
The total area of the figure = Area of the trapezoid ABEF + Area of the rectangle BCDE
Step: 2
Area of the trapezoid ABEF = (12) × height × (sum of the measures of the parallel sides)
Step: 3
= (12) × FO × (AF + BE)
Step: 4
= (12) × 1 × (4 +6)
  [From the figure, FO = 1 ft, AF = 4 ft and BE = 6 ft.]
Step: 5
= (12) × 1 × 10
  [Add 4 and 6 in the grouping symbol.]
Step: 6
= 5 ft2
  [Simplify.]
Step: 7
Area of the rectangle BCDE = length × width = BC × CD
Step: 8
= 1 × 6
  [From the figure, BC = ED = 1 ft and BE = CD = 6 ft.]
Step: 9
= 6 ft2
  
Step: 10
The total area of the figure = 5 + 6 = 11 ft2
  [Substitute the values.]
Correct Answer is :   11 ft2
Q15Find the area of the figure.

A. 90 cm2
B. 66 cm2
C. 80 cm2
D. 137 cm2

Solutions
Share 
Step: 1
  [Draw BF¯ ⊥ ED¯.]

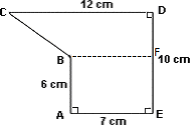
Step: 2
ABFE is a rectangle in which AB = EF = 6 cm and AE = BF = 7 cm. BCDF is a trapezium in which CD = 12 cm, BF = 7 cm and DF = 4 cm as shown.
Step: 3
Area of rectangle ABFE = 7 × 6 = 42 cm2
  [Area of a rectangle = length × width.]
Step: 4
Area of trapezoid = ( 12)(4)(7 + 12) = 38 cm2
  [Area of trapezoid = 12 h (b1 + b2).]
Step: 5
Total area of the figure = 42 + 38 = 80 cm2
Correct Answer is :   80 cm2

HighSchool Math