Power Properties
Definition Of Power Properties
Power of a Power Property: This property states that the power of a power can be found by multiplying the exponents.
That is, for a non-zero real number a and two integers m and n, (am)n = amn.
Product of Powers Property: This property states that to multiply powers having the same base, add the exponents.
That is, for a real number non-zero a and two integers m and n, am × an = am+n.
Quotient of Powers Property: This property states that to divide powers having the same base, subtract the exponents.
That is, for a non-zero real number a and two integers m and n,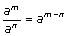
.
Power of a Product Property: This property states that the power of a product can be obtained by finding the powers of each factor and multiplying them.
That is, for any two non-zero real numbers a and b and any integer m, (ab)m = am × bm.
Power of a Quotient Property: This property states that the power of a quotient can be obtained by finding the powers of numerator and denominator and dividing them. That is, for any two non-zero real numbers a and b and any integer m,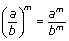
.
Video Examples: Power of a Power Property
Example of Power Properties
In the above figure, the letter R is on the top.
Power of a Power Property: (22)3 = 43 = 64 is the same as 22×3 = 26 = 64.
Product of Powers Property: 22 × 25 = 4 × 32 = 128 is the same as 22+5 = 27 = 128.
Power of a Product Property: (3 × 4)2 = 122 = 144 is the same as 32 × 42 = 9 × 16 = 144.
Quotient of Powers Property: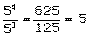
is the same as 54-3 = 51 = 5.
Power of a Quotient Property: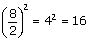 
is the same as
.
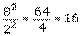Solved Example on Power Properties
Ques: Evaluate: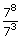 
Choices:
A. 823,543
B. 16,807
C. 2,401
D. 117,649
Correct Answer: B
Solution:
Step 1: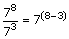 
[To divide powers with same base, subtract their exponents.]
Step 2: = 75 = 16,807 [Simplify.]
Step 3: So,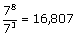 
.